We discussed Newton's Laws of Motion.

Newton's first law states that a body in (straight-line) motion tends to remain in motion and a body at rest tends to remain at rest unless acted upon by an external (and unbalanced) force. Vocabulary: we generally call the tendency to remain at rest "inertia" and the tendency to remain in motion "momentum". An object's momentum p is its mass m multiplied by its velocity v: p=mv

Newton's second law is typically associated with the formula F=ma and says that an object subject to a net force will accelerate. Actually Newton said the net force on a body is equal to the rate of change of momentum: F = dp/dt. The distinction arises in problems where mass is added to or taken away from the object of interest during the time under consideration -- for example something like a pickup truck during a hail storm.

Newton's third law states that for every action (force) there is an equal and opposite reaction. We used the example of a hand pushing (exerting a force on) a bottle, then the bottle in turn exerted a force on the hand (creating the pressure that lets the hand "know" the bottle is there).  It is important to note that these equal and opposite forces are on different objects; otherwise, one would find oneself in a situtaion where there was never any acceleration. 

Newton's law of universal gravitation states that every mass exerts a force of attraction on every other mass.  The magnitude of that force is proportional to each of the two masses in question and inversely proportional to the square of the distance between them -- where by the distance we mean the distance between their "centers". What makes this law "universal" is that it applies to all masses and is responsible both for apples falling from trees and the Moon orbiting around the Earth. If this is true then we should be able to recover from this law the results of Galileo (falling masses near the surface of the Earth experience the same acceleration, approximately 9.8 m/s²) and the results of Kepler. Combining Newton's second law and his law of universal gravitation, we find.

F = m_object a = - G M_Earth m_object / R_Earth²

where the minus sign implies attraction.  Note that both sides of the equation have m_object, so it will drop out when we solve for the acceleration. Using the following values

• Gravitational constant G = 6.67300 × 10^-11 m³ kg^-1 s^-2
• Mass of Earth M_Earth = 5.9742 × 10²⁴ kg
• Radius of Earth R_Earth= 6.3781 × 10⁶ m

We found -9.8 m/². Hence we recovered Galileo's results -- the independence of a falling body's acceleration on its mass and the particular value for the acceleration due to gravity.

The inverse square dependence of Newton's universal law of gravitation is strongly tied to Kepler's Laws. (Side note: Part of the animosity between Hooke and Newton was Hooke's claim that he had come up with the inverse square dependence first.) Kepler's Laws are

1. The planets' orbits around the sun are elliptical with the sun at one of the foci.
2. When the planet is closer to the sun it moves faster, when it is farther from the sun it moves slower such that the area "swept" out by equal times is constant. This is an example of a conservation law. In particular it is conservation of angular momentum.
   
   
   
   
3. The square of the orbit's period ("year") -- time to complete its orbital is directly proportional to the cube of the orbit's "semi-major axis".

We opted to recover a simplified version of Kepler's third law from Newton's universal law of gravity by considering the orbit to be circular and at a constant speed. Since velocity is a vector -- has magnitude (speed in this case) and a direction, we can have an acceleration by changing either the speed or the direction. In a simplified, circular path only problem, the acceleration is all about changing the direction. This type of acceleration goes ny the name centripetal.

We considered turning around a corner. If we proceed at a higher speed, we have a higher rate of change of velocity since we are making a bigger change and doing it in less time. If we take a turn with a larger radius, then the acceleration goes down since it will take longer to make the turn if it involves greater distances. After this argument we considered a dimensional (scaling) argument. Acceleration has units m/s², speed has units m/s and radius has units of m. We could make a quantity having the units of acceleration from the speed and radius with the formula a_centripetal varies as v²/r. A scaling argument does not tell one that the coeffecient is minus one, but it is. a_centripetal = - v²/r, where the minus sign is associated with the direction.

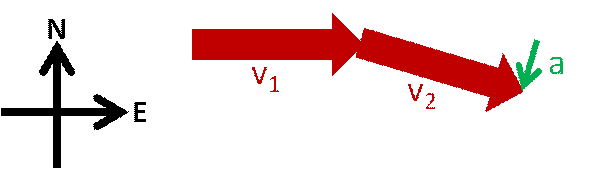
In the picture above we looked at the direction of the change in velocity, that is the direction of acceleration. With the velocity starting East and changing to South of East, the direction of acceleration is South and a little West (since the amount going East is slightly decreased). In general the centripetal acceleration is said to be towrd the center of the circle. Again, combining Newton's second law and his law of universal gravitation yields

F = m_planeta = -m_planet v²/r = -G M_sun m_planet / R_Sp²

where R_Sp is the distance between the Sun and the planet. The speed v is given by 2 π R_Sp / T, where 2 π R_Sp is the circumference (the length around the orbit) and T is the period (the time for one complete cycle).  Substituting the speed in and some cancelling yields

4 π² R_Sp³ = G M_sun T²

We finished by talking about conservation laws such as

• Momentum (where our example was a car crash)
• Angular momentum (where our example was an ice skater spinning -- but also demoed by me in a swivel chair)
• Mass and Energy, which used to be thought of as two separate conservation laws until Einstein came along with his E=mc² which is most prevalent in nuclear phenomena such as fission and fusion
• Charge