General Physics Lab: Conservation Of Momentum

PYL 105

Collision Carts and Conservation of Momentum

The momentum of an object is the product of its mass and its velocity.

p = m v

The bold letters are used above to convey that momentum, represented by the p, is a vector. In addition to the momentum of a single object, one can consider the momentum of a "system" – a combination of related objects.

P = p₁ + p₂ + ... = m₁ v₁ + m₂ v₂ + ...

The quantity P is useful because it is conserved (does not change with time) under certain circumstances. The circumstances are when there is no net external force on the system.

F_net,ext = 0

By "external" we mean forces exerted by bodies that are not part of the system on bodies that are part of the system. The claim is that internal forces, forces exerted by one body in the system on another, do not affect P, the momentum the system.

Find the mass of two collision cars and two metal blocks and enter them in the table provided below. Your collision cars should have ends with Velcro patches.
Set up a motion sensor (with a frequency of 20 Hertz) at the end of a track.
Place Cart 1 near the end of the track with the motion sensor (but about 50 cm away) and Cart 2 further down the track, as shown below.
The Velcro ends should be facing each other, so that the carts will stick together when they collide. (The carts with the green stickers have a magnet in them. You can use one green-sticker cart, but you cannot use two green-sticker carts.)
Start recording and give Cart 1 a quick push.
Our analysis will be based on the assumption that the carts stick together. Throw out the run if this was not the case.
Make a plot of the velocity versus time data eliminating any bad data at the beginning and end of recording. It should look something like the graph shown below. (Include all of the graphs in your report.)
The sharp fall off in velocity (around t=2.4 s in the graph above) occurs when the carts collide and stick together. Find the velocity of Cart 1 just before the collision and the velocity of the Cart 1/Cart 2 combination just after the collision. Record these in the table provided below.
Calculate the momentum of Cart 1 just before the collision and just after the collision. Also calculate the momentum of Cart 2 just after the collision. Enter them in the table provided below.
Calculate the momentum of the system just before and just after the collision as well as the percent difference and enter them in the table provided below.

Repeat the measurements and calculations for the following cases:

trial 2: add one block to Cart 1.
trial 3: add a second block to Cart 1.
trial 4: remove the blocks from Cart 1; add one block to Cart 2.
trial 5: add a second block to Cart 2.

Don't forget to include the mass of the block(s) in your calculation of momentum.

DATA AND CALCULATIONS

Masses

Item	Cart 1	Cart 2	Block 1	Block 2
Mass (kg)

Velocities

trial	Velocity before Cart 1 (m/s)	Velocity after Carts 1 & 2 (m/s)
1
2
3
4
5

Individual momenta

trial	Momentum before Cart 1 (kg m/s)	Momentum after Cart 1 (kg m/s)	Momentum after Cart 2 (kg m/s)
1
2
3
4
5

Momentum of the system

trial	Momentum before System (kg m/s)	Momentum after System (kg m/s)	Percent difference
1
2
3
4
5

The change in mometum is equal to the "impulse", the force integrated over time.

I = p_final - p_initial

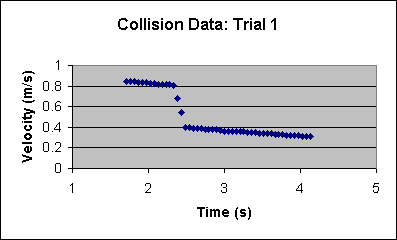
We can find the average force exerted on Cart 1 by Cart 2 during the collision by taking the change in momentum of Cart 1 and dividing it by the duration of the collision.

F_ave = I / D t

We can estimate the duration by examing the velocity versus time graph made earlier. Take the last point of the upper line and the first point of the lower line and finding the difference in the two times.

Impulse and average force

trial	Impulse Cart 1 (kg m/s)	Duration (s)	Force of Cart 2 on Cart 1 (N)
1
2
3
4
5

There are external forces acting on the two-cart system. What are they?