PYL 105

Conservation of Energy

Much of our previous studies have been about how various quantities change; a velocity is the rate of change of position; an acceleration is the rate of change of velocity; a net force is the cause of an acceleration. However, now we consider a quantity that at least under certain circumstances does not change. The energy of an "isolated" system is conserved meaning that the system's energy does not change as a function of time. Just what is and what isn't a part of the system can be an important decision in considering such problems. Consider the following constant-acceleration equation

v² = v₀² + 2 a ( x - x₀)

The above equation does not involve time and using it is essentially an energy analysis. The velocity-squared terms are associated with kinetic energy (the energy associated with an object that is in motion). The acceleration times displacement term can be associated either with the concept of work (which is how an external force in a non-isolated system can change the system's energy) or the concept of potential energy (the energy associated with an arrangement of objects within the system).

An Energy Analysis of Atwood's machine

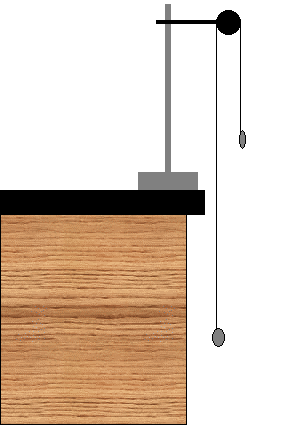
The figure below shows an Atwood's machine, two unequal masses (m₁ and m₂) connected by a string that passes over a pulley.

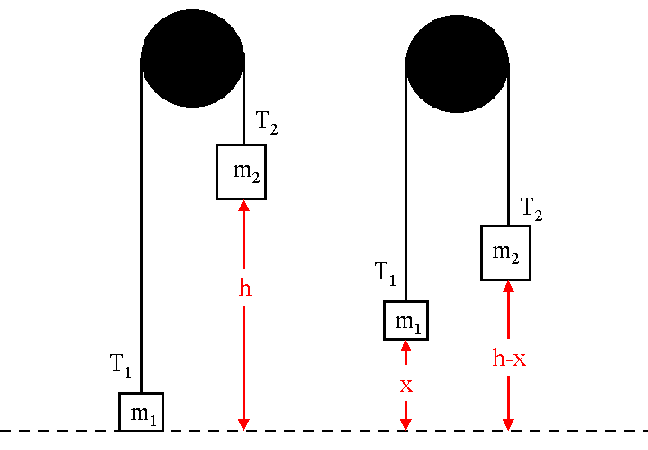
Consider the forces acting on each mass. Assume that the string is massless and does not stretch and that pulley is massless and frictionless. Derive an expression for the acceleration; it should have the form

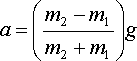
Of course, this is an idealized calculation, and we cannot expect to find this acceleration experimentally. Let us keep the other approximations but drop the frictionless approximation, identifying friction as the cause of any deviation from the ideal acceleration given above. We can then relate the frictional force to the difference between the ideal and experimental accelerations. The ideal force leads to the ideal acceleration

F_ideal = (m₁+m₂)a_ideal

Including the frictional force leads to the experimental acceleration

F_ideal - F_fric = (m₁ + m₂) a_exp

Solve these for F_fric.

We could also adopt an energy approach to this problem. One of the first steps in the energy approach is to determine the "system" for which we are calculating the energy. The "system" could be

• m₁ alone
• m₁ and the earth
• m₁, m₂ and the earth

Determining the system determines whether a force is identified as external or internal and in some cases whether we talk about work or potential energy. For the systems above

• gravity and tension are external
• gravity is internal, tension is external
• gravity and tension are internal

If we adopt the last choice m₁, m₂, and the earth as our system, then we have kinetic energy of the two blocks and gravitational potential energy of the two blocks. Friction involves the string rubbing against the pulley and the blocks moving through air (and possibly the microscopic motions and arrangemnts of atoms within the blocks) and remains external to our system. Ignoring friction for the moment we would find

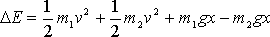
where x is the displacement of the blocks -- one moving with the gravitational force and the other against it. This result is the energy of the m₁-m₂-earth system and ideally ΔE=0, that is, the energy of the m₁-m₂-earth system is conserved. That is, ideally the energy does not change from its initial value which can be taken to be

E₀ = m₂ g h

again assuming that the system starts from rest in the position shown on the left above.

Of course, friction must be brought into the energy approach as well. Just as friction accounted for the deviation from the ideal acceleration in the previous approach, it should also account for any changes in the energy in the latter approach. That is, the work associated with the frictional force (the work done against the frictional force) should equal the observed change in energy.

Measurements

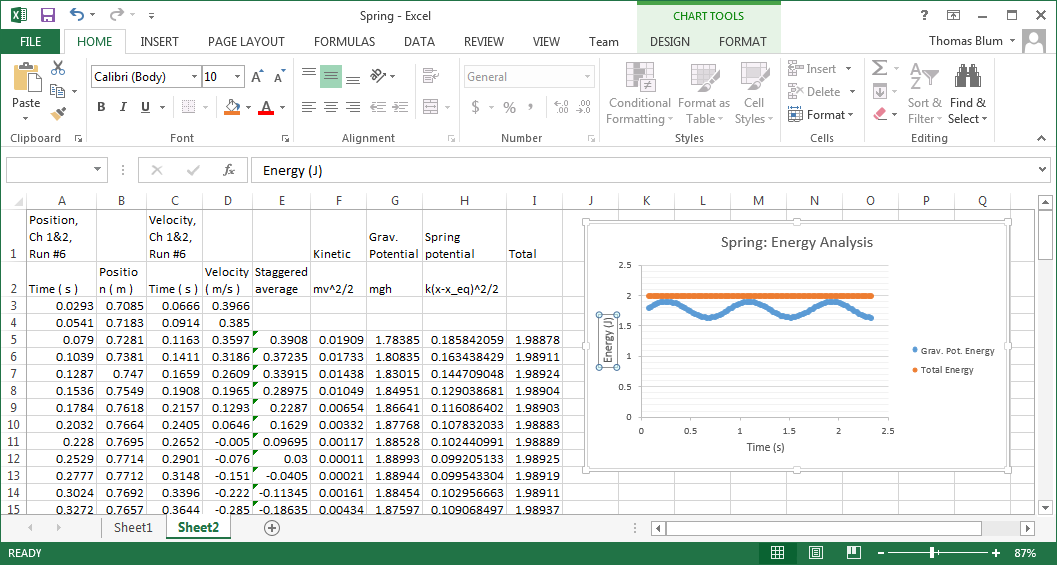
• Find the masses of two sinkers. Use two that are not the same size but are close in size. (The calculations are sensitive to these mass measurements, make them carefully.)
  
  

  m1	m2	h

  
  
• Set up an Atwood's machine, two uneven masses connected by a string that passes over a smart pulley. Set it up at the edge of the bench as shown below so that we can extend our experimental range.
  
  
  
  
  
  
• Set up the interface to use the Smart Pulley.
• Hold the lighter sinker down at the floor level, note the height of the heavier sinker, start recording data and release.
• Copy and paste both the position versus time and velocity versus time data over to Excel. Plot velocity versus time.
• Recall that we do not want all of this data. At some point the lower sinker hits the ground or the higher sinker gets caught in the pulley. Toss out the undesired large-time data.
• Fit a new graph (with bad data thrown away) to a line and extract the experimental acceleration.
• Compare this acceleration to the ideal one and calculate the frictional force
  
  

  aideal     ( )	aexp      ( )	Ffric      ( )

  
  
  
• Note that the times associated with the position data and the times associated with the velocity data are different. The times are staggered.

  

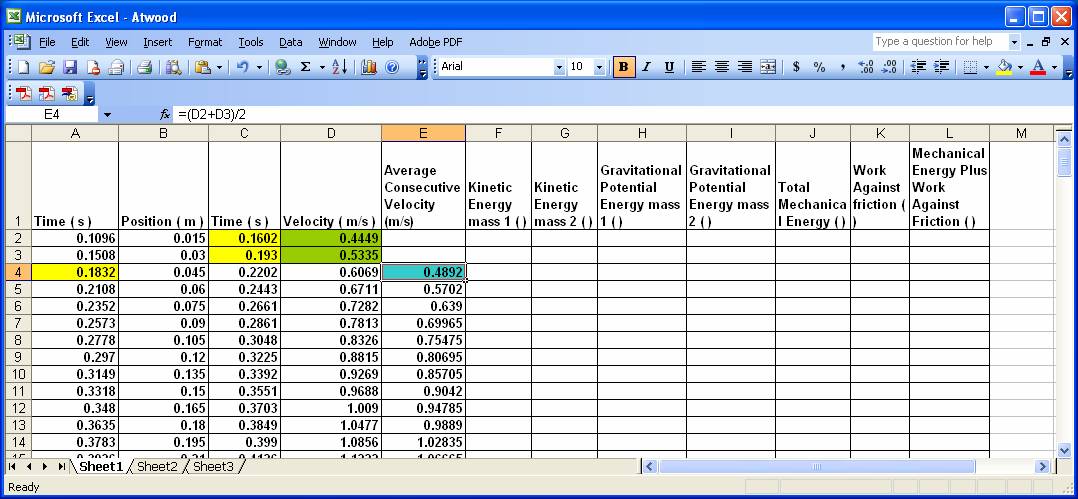
  Calculate the average of two consecutive velocities. For example, you might enter a formula like

  = (D2 + D3)/2

  in cell E4. This will determine the velocities at roughly the times for which we have position data.
• Next use Excel to calculate the terms in the mechanical energy. Use the velocity just obtained.
• kinetic energy of first mass (m₁v²/2)
• kinetic energy of second mass (m₂v²/2)
• gravitational potential energy of first mass (m₁gx)
• gravitational potential energy of second mass (m₂g(h-x))

(In the formulas above m₂ was the heavier mass and started at the higher position.) The corresponding Excel formula could be placed in columns F,G,H and I as shown above.

• In the next column (J), sum these four energy terms. This result is the mechanical energy. Plot mechanical energy versus (position) time.
• If there were no friction this would be a horizontal line (why?)
• How much energy is lost?
• If the change in energy is due solely to the presence of a frictional force, then we can calculate the work done against a constant friction as follows

  W_fric = F_fric x

  Calculate the work done against friction (in column K).

• Add the work done against friction to the mechanical energy. Make a graph which plots simultaneously mechanical energy versus time and mechanical energy plus work done against friction versus time. (Highlight three columns: the time column, the mechanical energy column and the mechanical energy plus work done against friction column, go to Chart Wizard and proceed as usual.) The second curve should be noticeably flatter than the first. Why?
• Is all energy now accounted for?

Hooke's Law

An object is elastic if

• it is deformed when a force is applied, and
• it returns to its origin shape when the force is removed

Our example of an elastic object will be a spring. You can apply a force that stretches or compresses it. The force that opposes the applied force is called the restoring force. If the restoring force is proportional to the amount of deformation, the object is said to obey Hooke's law.

One can study a spring from a force point of view or from an energy point of view.  We will look at a static spring from a force point of view and a moving (kinetic) case from an energy point of view.

Static Case

• Using a tall ringstand, clamps, etc. set a spring hanging vertically as shown below. We are using the tall ringstand so that the hanger does not get "too close" to the motion sensor

  

• When setting up the motion sensor, select a trigger rate of 40 Hz (put it back to lower setting if the data looks bad -- if the lengths do not match up with a quick meterstick measurement).
• We will apply known weights to the hanger and note the displacement using the motion sensor for consistency with the next part of the experiment. Record a second or so of position data with the motion sensor and use the Sigma button and copy down the mean position.
• It is not convenient to measure displacement x. What we will measure instead is L, the distance from the bottom of the hanger to a motion sensor on the lab table.
• It is crucial that you understand that this length L is not the displacement x that appears in Hooke's law

  F = - k x,

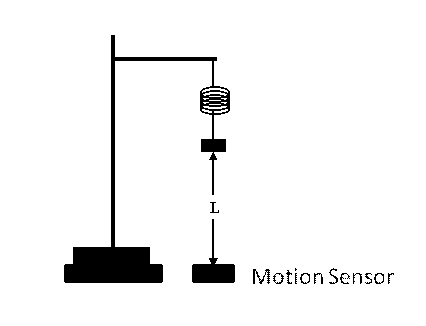
  However, L and x are related. What is the relationship? (Note that even with the just the hanger, there is a bit of displacement from the weight of the hanger. You may need to make a graph before you can fill in the displacement column below.)

• Add masses to the hanger: 50 g, 100 g, 150 g, 200 g and 250 g and note the distance L.

• Calculate the "applied forces" (the weights, hanger included) and make a plot of Applied Force versus L.
• Fit it to a straight line.
  • What is the interpretation of the slope?
  • What is the interpretation of the x intercept?
  • What is the interpretation of the y intercept?
  (One of these intercepts is the equilibrium position -- the position from which the spring's stretched distance is measured)
  
• Find the x's (displacements) that correspond to your L's and fill in the table above.
• The proportionality constant k is called the spring constant or sometimes a force constant. What is the spring constant for this spring? What are the units (dimensions) of the spring constant?
• What is the meaning of the minus sign in Hooke's law?

Kinetic Case

• Pull one of the larger masses down a couple centimeters and release.
• Record the motion.
• Build an Excel spreadsheet similar to the one for the Energy Analysis of the Atwood Machine. There should be a number of rows with each row containing: position time, position, velocity time, velocity. Make the adjustments (like last time) so that velocity and position are measured at APPROXIMATELY the same time, calculate all kinetic energy terms, all potential energy terms, total mechanical energy.
• Note that the gravitational potential energy (mgh) can be measured relative to "any" reference point -- use the motion detector's postion, BUT the spring's potential energy kx²/2 must be measured relative to its equilibrium position k(x-x_eq)²/2. (x_eq was found above.)
• Make a plot of position versus time and velocity versus time (2 items on one graph).
• Make a plot of total energy versus time and gravitational potential energy versus time (2 items on one graph). Comment on the relative variation in energy you see for the total energy compared to just the gravitational potential energy.